Volume 17, Number 1, Jan 1995, p.19

The Determination of Allowable RH Fluctuations

This and the following three articles all address the subject of climate control guidelines in museums. These articles are drawn from different sources and were not written in response to each other. The first piece by David, which was solicited by the Newsletter, was written as an overview of the CAL research and was never intended to address the issues criticized in the articles by Will Real and William Lull. Had publication deadlines allowed, Dr. Erhardt would have wished to clarify what he considers to be misunderstandings and misinterpretation of some of CAL's data in these articles. The second article is taken from the December 1994 issue of The Torch, a monthly publication for Smithsonian employees. It is not the press release referred to in the following two pieces (the press release is printed in full in the Abbey Newsletter, Aug.-Sept. 1994, v.18 #4-5), but contains substantially the same information. Finally, the last two articles, reprinted from the November 1994 Abbey Newsletter, were chosen because they provide opinions from two different segments of the conservation community.

This is, obviously, a subject of great concern to conservation and one about which thoughtful professionals have not reached a consensus. It is hoped that presentation of these articles will encourage constructive discussion.

The most important factor in the preservation of collections is the maintenance of proper environmental conditions. Many environmental factors such as temperature, relative humidity (RH), light, pollution, and vibration affect the permanence of objects and materials. This article is an introduction to work that we have conducted relating to the determination of allowable fluctuations in RH.

Several considerations are involved in specifying RH for climate control. The first is the RH setpoint, the value that you are trying to maintain. The second is the allowable fluctuation, the short term variation that will be allowed. Third is the seasonal drift that will be allowed, the amount by which the setpoint is allowed to vary over the year. It is possible, if the allowable fluctuations are large enough, that seasonal drift might be accommodated within one overall allowable range.

A discussion of allowable humidity fluctuations should begin with a short summary of how the present guidelines developed. It has been known for a very long time that the extremes of RH cause damage. High RH results in mold growth and softening, while excursions to low RH can cause cracking and fracture sensitivity. Changes in RH were blamed for such environmentally induced damage as flaking paint, cracked wood, and glue failure. Reports of such damage increased as central heating became more widespread. Simple experiments in which some types of damage were duplicated by subjecting materials to large swings in RH showed that it was not just specific values of RH but changes in RH that could cause damage.

Anecdotal evidence indicated that damage could be prevented or minimized by maintaining a constant, moderate RH. The most famous example is that of the collections of the National Gallery, London. During World War II, the Gallery's collections were moved for safekeeping to mines in Wales. The climate in the caves was constant, although at too high an RH. The RH was adjusted by slightly heating the air to maintain between 55 and 60% RH, which earlier experiments had shown was the effective average in the then un-air conditioned Gallery. Within a matter of months, cracking, flaking, and other such problems that had occurred in the Gallery essentially disappeared. The problems returned when the collections were returned to the Gallery after the war. This experience was a prime justification for installing climate control soon after.

Obviously, much of the benefit of maintaining a constant, moderate RH derives from the avoidance of damaging extremes of RH. Benefit also was thought to derive from the avoidance of even small fluctuations, since large fluctuations were known to cause damage. Experiences such as that at the Gallery, combined with a lack of knowledge of whether small changes in RH caused damage, led to the present goal of maintaining a constant RH, or "flatlining". One value of RH was maintained year-round, with both rapid or daily changes (fluctuations) and seasonal changes (drift) reduced to the extent possible. Specifications often exceeded the ability of equipment or buildings to cope. This led to a number of problems. Overengineering of HVAC systems was common, often requiring major changes in the fabric of historic structures. Most older buildings, and surprisingly many new ones, are not capable of maintaining 50% or higher RH during very cold weather without condensation occurring in the building fabric. Maintaining one specific value of RH year-round can be much more expensive than allowing a seasonal drift, or bypassing RH control altogether when outside (intake) air is within a specified humidity range.

Practically, few institutions achieve the perceived "optimum" of absolutely constant RH. Most either allow seasonal drift or tolerate larger fluctuations than could be achieved with unlimited budgets. This is done, though, with a vague sense that the climate is not perfect, and that some tradeoff is being made in allowing some damage, however small, to occur and accumulate. Many get by with outdated or inefficient equipment, waiting until they can afford to install and keep up a system capable of achieving environmental nirvana. Buffered display cases, microclimates, and other techniques are used extensively to improve upon ambient conditions thought to be less than optimal.

Obviously, things would be much simpler if the environmental requirements could be relaxed without causing damage. This requires answering two basic questions. First, do all RH fluctuations, no matter how small, cause damage, or is there a threshold of allowable RH fluctuation below which there is no damage? Second, if some fluctuation is allowable, how much? Additional questions can be asked, such as whether damage depends upon the rate of RH change, but research capable of answering the first two questions should provide most answers.

Our approach has been to determine the mechanism of damage caused by RH fluctuations, and the properties of materials involved in processes leading to damage. Many materials such as wood, glue, and paint absorb and desorb water and consequently change dimension as the relative humidity changes. If a material is unrestrained, this absorption and desorption is reversible within a reasonable range of relative humidity, and a material simply expands and contracts with changes in relative humidity. It is only when a material is restrained, either internally or externally, that this tendency to change dimension can cause stresses and resulting damage. If we lower the relative humidity, wood will try to shrink. If it is held in a rigid metal frame and prevented from shrinking, stresses develop. If these stresses are large enough, they result in permanent deformation or breakage. The question now becomes: Is there a range in which a material can be reversibly deformed, and how can one determine the relationship between RH and these stresses?

Figure 1. Stress-strain curves at various relative humidities for cottonwood in the tangential direction. The curves are displaced on the X axis by the change in dimension due solely to differences in RH.

Figure 1 shows stress-strain curves for a piece of cottonwood at various relative humidities. These tests were conducted tangential to the grain, the weakest and most RH sensitive direction. Such data is typical for the many materials that we have tested. Applying a force (moving up the vertical axis) stretches the sample (moving to the right along the horizontal axis). Changes in dimension also can be produced simply by changing the RH with no force involved. This involves moving along the horizontal axis, which is why the stress-strain curves for different values of RH start at different positions along the horizontal axis. The curves are separated by the change in length due solely to changes in RH. The beginning of each curve is linear, and in fact is reversible. If we stay within the linear section of a curve, the wood resumes its original shape when the force is released. It is only when changes in length exceed certain values that the wood is irreversibly deformed (yields), or eventually breaks. The stress-strain curves allow us to determine how much a sample can be stretched without causing damage. For most materials, this value is about 0.3-0.4% of the original length.

Figure 2. Detail of Figure 1. A sample of cottonwood held at constant length while the RH is lowered from 48 to 23% RH develops the same stress as a sample which is allowed to shrink while drying and then stretched to the original length.

How do we relate these tests, conducted at constant RH, with effects caused by changes in RH? The answer is illustrated in Figure 2, a detail of Figure 1. Assume the worst case, that a sample is fully restrained and not allowed to change dimension. If the RH is lowered from 48%, the sample tries to shrink, but cannot. Stress develops. This is equivalent to moving vertically, staying the same length but with increasing stress. If we reduce the RH to 23%, we end up at a point on the 23% RH stress-strain curve! This demonstrates a fundamental principle we have found to be true for all of the materials we have tested. Each point on the stress-strain curve corresponds to a unique RH. In other words, the path you take to a certain condition does not matter. Keeping the dimension constant and lowering the RH results in the same stress as allowing the sample to shrink freely as the RH is lowered, and then stretching it to the original length. This means that the effects of RH changes can be calculated from a series of stress-strain tests conducted at constant, but different, values of RH, rather than having to run much more time consuming tests involving fluctuating RH values. The only additional information required is the change in dimension caused by changes in RH. If we know the change in dimension caused by RH changes, and the amount a sample can be reversibly stretched, then we can calculate the allowable RH fluctuation.

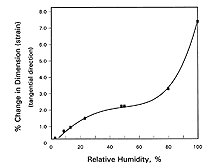Figure 3. Moisture absorption isotherm for cottonwood. The change in dimension in the tangential direction is plotted as a function of RH.

Figure 4. Swelling coefficient for cottonwood (tangential direction) as a function of RH. Cottonwood is relatively unresponsive to RH changes in the 40-60% RH range.

Figure 3 is the moisture absorption isotherm for cottonwood, a plot of the change in length in the tangential dimension as a function of RH. The slope of this curve is therefore a measure of the sensitivity of dimension to changes in relative humidity. For a specific range of RH, the flatter the curve the smaller the response to changes in RH and the greater the change in RH required to cause damage. Figure 4 is a plot of the slope of the isotherm, the response to RH change. We see that the response to change in RH is least in the moderate RH range, and greatest at high and low RH. In the middle region, large changes in relative humidity must take place before dangerous stresses are possible. Thus, the allowable fluctuation in relative humidity is greatest in the 40-60% RH range, and least at the RH extremes. Changes in RH required to cause irreversible deformation, or ultimately failure, can be calculated for each RH.

Figure 5. Plot of RH values which produce yielding or failure in restrained cottonwood (tangential direction) as a function of equilibrium RH value. A restrained, stress-free sample at 50% RH can be raised or lowered to 68 or 31% RH without permanent deformation. Failure occurs below 13% RH.

This information is illustrated in Figure 5. This figure plots RH values which produce yield and failure as a function of the equilibrium, or stress-free, RH. These values assume full restraint. Starting at 50% RH, for example, one can reduce the RH to about 31% before yield, or irreversible deformation, starts to occur, and to about 13% before it breaks. Alternatively, the RH can be raised to about 68% before yielding in compression (compression set). The wood tries to expand, but is held "compressed" to its original length. Failure in compression is a more complex phenomenon than breaking in tension, and is not considered here. Thus, for cottonwood at equilibrium at 50% RH, the allowable RH fluctuation is at least +18% RH. Remember, these calculations are for the worst case, tangential to the grain and assuming full restraint. An unrestrained sample can reversibly swell and shrink over much larger RH ranges.

Note the very small allowable ranges at high and low RH. The dimensional response to RH changes increases at the extremes of RH, but the allowable reversible dimensional change does not. A restrained piece of cottonwood conditioned to outside conditions of over 70% RH cannot be brought into a museum at 50% RH without damage. This data also refutes the idea that high RH avoids mechanical damage because materials are softer and more flexible. Damage occurs, but in a different way. Materials are less likely to fracture, but permanent deformation does occur.

We have carried out such measurements and calculations for many other materials. Several points of interest emerge. The allowable fluctuation is a function of the relative humidity to which the object is equilibrated. The relative humidity with the maximum allowable fluctuation varies with the material. And, the allowable fluctuations can be quite different for different materials. An important point is that all of the allowable fluctuations are larger than those generally presently recommended, even though these values are extremely conservative. These values assume full restraint of the materials, long term exposure to the RH extremes, and produce changes that are well within the reversible, elastic range.

These values apply to individual materials. Because the allowable dimensional changes for most materials fall within the same range, the same limits also apply directly to composite objects. As long as you stay within the allowable range of the most sensitive material present, then no excessive strains will be produced in any material of the object.

Composite objects may, in fact, behave much like a single material. If all the materials have approximately the same dimensional response to RH changes, then the entire object swells and shrinks without producing significant stresses. The exception, of course, is massive objects in which the exterior responds to RH changes before the interior. In this case, the interior acts as an internal restraint, and the stresses and allowable fluctuations are as calculated for the restrained material. The rate of change of RH is not critical, as long as the maximum allowable strains are not exceeded.

Figure 6. Swelling coefficients for typical layers of a panel painting as a function of RH. The layers respond similarly at moderate RH, but the wood (tangential direction) and glue layers shrink and expand much more than the gesso and paint layers at high and low RH. The wood has negligible response along the grain.

A good example of a composite object is a painting on a wood panel. Figure 6 plots the RH sensitivity of the components of the layers of a typical panel painting. The values for cottonwood are plotted for the cross-grain direction only. Its response along the grain is so low as to be negligible. Because the wood panel is so thick relative to the other layers, its response determines the dimensional change (or lack of it) in the other layers. Cottonwood has an extremely small dimensional response to changes in RH along the grain, and essentially acts as a restraint. High RH produces compression in the upper layers in the grain direction as they try to expand, and low RH produces tension as they try to shrink. Across the grain, the situation is very different. In this direction, the RH sensitivity of the wood is about the same as the other materials at moderate RH. Changes in the middle RH range produce little stress, since all of the layers respond similarly. At high RH, however, the response of the wood increases dramatically, and the wood expands faster than the other layers. The wood can actually stretch the other layers at high RH! Similarly, at low RH, the greater shrinkage of the wood can result in compression of the gesso and paint layers. Stresses, strains, and resulting allowable RH fluctuations of composite objects all can be calculated directly from data such as that presented here.

Mechanical damage due to RH changes is not the only consideration in determining appropriate environmental conditions. We presently are in the process of similar research on the effects of temperature. Other factors, such as chemical reactivity, corrosion processes, hygroscopic salts, etc., also come into play. Such considerations were addressed in a paper presented at the recent IIC congress in Ottawa [1].

That most museum objects can tolerate, without mechanical damage, larger fluctuations than previously thought is not an excuse to abandon climate control. To the contrary, there always will be some materials and objects that require conditions different from or more tightly controlled than the main collection [1]. Standard approaches such as the use of microclimates and buffered cases are appropriate for such exceptions. If anything, the relaxation of allowable RH fluctuations for the general environment requires more thought and a better knowledge of the materials, history, and requirements of the collection.

This work is one result of a number of collaborative research projects related to environmental conditions in the museum that are being conducted by the authors at the Conservation Analytical Laboratory of the Smithsonian Institution. David Erhardt conducts research on the effects of environmental conditions on chemical degradation processes, Marion F. Mecklenburg specializes in structural mechanics, Charles S. Tumosa in materials properties, and Mark McCormick-Goodhart in environmental conditions for the storage of photographic materials. Questions or comments about our work can be directed to us at the following numbers and address:

Reference

1. David Erhardt and Marion Mecklenburg, "Relative Humidity Reconsidered", Preventive Conservation: Practice, Theory and Research, Preprints of the Contributions to the Ottawa Congress, 12-16 September 1994, The International Institute for Conservation of Historic and Artistic Works, pp. 32-38.