Solubility Parameters: Theory and Application
John BurkeIt is the total van der Waals force, however, which is reflected in the simplest solubility value: the Hildebrand solubility parameter. The solubility parameter is a numerical value that indicates the relative solvency behavior of a specific solvent. It is derived from the cohesive energy density of the solvent, which in turn is derived from the heat of vaporization. What this means will be clarified when we understand the relationship between vaporization, van der Waals forces, and solubility.
When a liquid is heated to its boiling point, energy (in the form of heat) is added to the liquid, resulting in an increase in the temperature of the liquid. Once the liquid reaches its boiling point, however, the further addition of heat does not cause a further increase in temperature. The energy that is added is entirely used to separate the molecules of the liquid and boil them away into a gas. Only when the liquid has been completely vaporized will the temperature of the system again begin to rise. If we measure the amount of energy (in calories) that was added from the onset of boiling to the point when all the liquid has boiled away, we will have a direct indication of the amount of energy required to separate the liquid into a gas, and thus the amount of van der Waals forces that held the molecules of the liquid together.
It is important to note that we are not interested here with the temperature at which the liquid begins to boil, but the amount of heat that has to be added to separate the molecules. A liquid with a low boiling point may require considerable energy to vaporize, while a liquid with a higher boiling point may vaporize quite readily, or vise versa. What is important is the energy required to vaporize the liquid, called the heat of vaporization. (Regardless of the temperature at which boiling begins, the liquid that vaporizes readily has less intermolecular stickiness than the liquid that requires considerable addition of heat to vaporize.)
From the heat of vaporization, in calories per cubic centimeter of liquid, we can derive the cohesive energy density (c) by the following expression
![]()
where:
c=Cohesive energy density
h=Heat of vaporization
r=Gas constant
t=Temperature
vm=Molar volume
In other words, the cohesive energy density of a liquid is a numerical value that indicates the energy of vaporization in calories per cubic centimeter, and is a direct reflection of the degree of van der Waals forces holding the molecules of the liquid together.
Interestingly, this correlation between vaporization and van der Waals forces also translates into a correlation between vaporization and solubility behavior. This is because the same intermolecular attractive forces have to be overcome to vaporize a liquid as to dissolve it. This can be understood by considering what happens when two liquids are mixed: the molecules of each liquid are physically separated by the molecules of the other liquid, similar to the separations that happen during vaporization. The same intermolecular van der Waals forces must be overcome in both cases.
Since the solubility of two materials is only possible when their intermolecular attractive forces are similar, one might also expect that materials with similar cohesive energy density values would be miscible. This is in fact what happens.
In 1936 Joel H. Hildebrand (who laid the foundation for solubility theory in his classic work on the solubility of nonelectrolytes in 1916) proposed the square root of the cohesive energy density as a numerical value indicating the solvency behavior of a specific solvent.
![]()
It was not until the third edition of his book in 1950 that the
term "solubility parameter" was proposed for this value and
the quantity represented by a delta (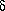 ). Subsequent authors
have proposed that the term hildebrands be adopted for
solubility parameter units, in order to recognize the tremendous
contribution that Dr. Hildebrand has made to solubility theory.
). Subsequent authors
have proposed that the term hildebrands be adopted for
solubility parameter units, in order to recognize the tremendous
contribution that Dr. Hildebrand has made to solubility theory.
Table 1 lists several solvents in order of increasing Hildebrand parameter. Values are shown in both the common form which is derived from cohesive energy densities in calories/cc, and a newer form which, conforming to standard international units (SI units), is derived from cohesive pressures. The SI unit for expressing pressure is the pascal, and SI Hildebrand solubility parameters are expressed in mega-pascals (1 mega-pascal or mpa=1 million pascals). Conveniently, SI parameters are about twice the value of standard parameters:
/cal1/2cm-3/2 = 0.48888 x
/MPa1/2
(3) /MPa1/2 = 2.0455 x
/cal1/2cm-3/2
(4)
Literature published prior to 1984 should contain only the common
form, designated 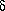 , and it is hoped that where the
newer SI units are used, they are designated as such, namely
, and it is hoped that where the
newer SI units are used, they are designated as such, namely 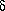 /MPa1/2 or
/MPa1/2 or 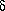 (SI). Obviously, one
must be careful to determine which system of measurement is being
used, since both forms are called Hildebrand parameters. This paper
will primarily use the SI values, and the use of standard values
will be noted.
(SI). Obviously, one
must be careful to determine which system of measurement is being
used, since both forms are called Hildebrand parameters. This paper
will primarily use the SI values, and the use of standard values
will be noted.
| Solvent | 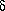 |
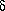 (SI) (SI) |
|---|---|---|
| n-Pentane | (7.0) | 14.4 |
| n-Hexane | 7.24 | 14.9 |
| Freon® TF | 7.25 | |
| n-Heptane | (7.4) | 15.3 |
| Diethyl ether | 7.62 | 15.4 |
| 1,1,1 Trichloroethane | 8.57 | 15.8 |
| n-Dodecane | 16.0 | |
| White spirit | 16.1 | |
| Turpentine | 16.6 | |
| Cyclohexane | 8.18 | 16.8 |
| Amyl acetate | (8.5) | 17.1 |
| Carbon tetrachloride | 8.65 | 18.0 |
| Xylene | 8.85 | 18.2 |
| Ethyl acetate | 9.10 | 18.2 |
| Toluene | 8.91 | 18.3 |
| Tetrahydrofuran | 9.52 | 18.5 |
| Benzene | 9.15 | 18.7 |
| Chloroform | 9.21 | 18.7 |
| Trichloroethylene | 9.28 | 18.7 |
| Cellosolve® acetate | 9.60 | 19.1 |
| Methyl ethyl ketone | 9.27 | 19.3 |
| Acetone | 9.77 | 19.7 |
| Diacetone alcohol | 10.18 | 20.0 |
| Ethylene dichloride | 9.76 | 20.2 |
| Methylene chloride | 9.93 | 20.2 |
| Butyl Cellosolve® | 10.24 | 20.2 |
| Pyridine | 10.61 | 21.7 |
| Cellosolve® | 11.88 | 21.9 |
| Morpholine | 10.52 | 22.1 |
| Dimethylformamide | 12.14 | 24.7 |
| n-Propyl alcohol | 11.97 | 24.9 |
| Ethyl alcohol | 12.92 | 26.2 |
| Dimethyl sulphoxide | 12.93 | 26.4 |
| n-Butyl alcohol | 11.30 | 28.7 |
| Methyl alcohol | 14.28 | 29.7 |
| Propylene glycol | 14.80 | 30.7 |
| Ethylene glycol | 16.30 | 34.9 |
| Glycerol | 21.10 | 36.2 |
| Water | 23.5 | 48.0 |
In looking over Table 1, it is readily apparent that by ranking solvents according to solubility parameter a solvent "spectrum" is obtained, with solvents occupying positions in proximity to other solvents of comparable "strength". If, for example, acetone dissolves a particular material, then one might expect the material to be soluble in neighboring solvents, like diacetone alcohol or methyl ethyl ketone, since these solvents have similar internal energies. It may not be possible to achieve solutions in solvents further from acetone on the chart, such as ethyl alcohol or cyclohexane--liquids with internal energies very different from acetone. Theoretically, there will be a contiguous group of solvents that will dissolve a particular material, while the rest of the solvents in the spectrum will not. Some materials will dissolve in a large range of solvents, while other might be soluble in only a few. A material that cannot be dissolved at all, such as a crosslinked three-dimensional polymer, would exhibit swelling behavior in precisely the same way.
It is an interesting aspect of the Hildebrand solvent spectrum that the Hildebrand value of a solvent mixture can be determined by averaging the Hildebrand values of the individual solvents by volume. For example, a mixture of two parts toluene and one part acetone will have a Hildebrand value of 18.7 (18.3 x 2/3 + 19.7 x 1/3), about the same as chloroform. Theoretically, such a 2:1 toluene/acetone mixture should have solubility behavior similar to chloroform. If, for example, a resin was soluble in one, it would probably be soluble in the other. What is attractive about this system is that it attempts to predict the properties of a mixture a priori using only the properties of its components (given the solubility parameters of the polymer and the liquids); no information on the mixture is required.
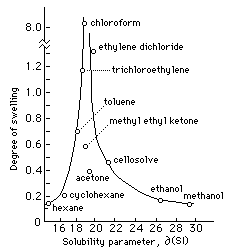
Fig. 1 Swelling of Linseed Oil Film in Solvents Arranged
According to Solubility Parameter (adapted from Feller, Stolow, and
Jones, On Picture Varnishes and Their Solvents)
Figure 1 plots the swelling behavior of a dried linseed oil film in various solvents arranged according to Hildebrand number. Of the solvents listed, chloroform swells the film to the greatest degree, about six times as much as ethylene dichloride, and over ten times as much as toluene. Solvents with greater differences in Hildebrand value have less swelling effect, and the range of peak swelling occupies less than two hildebrand units. By extension, we would expect any solvent or solvent mixture with a Hildebrand value between 19 and 20 to severely swell a linseed oil film. (The careful observer will notice certain inconsistencies in Fig. 1 which will be discussed later.)
Since a polymer would decompose before its heat of vaporization could be measured, swelling behavior is one of the ways that Hildebrand values are assigned to polymers (the general term cohesion parameter is often preferred to the term solubility parameter when referring to non-liquid materials). Another method involves cloud-point determinations in which a resin is dissolved in a true solvent and titrated with another solvent until the mixture becomes cloudy, thus identifying the range of solubility. Testing cloud-points with a variety of solvents and diluents enable a precise determination of cohesion parameter values for polymers. Other methods include a combination of empirical tests, such as cloud-point and solubility/swelling tests, with the addition of theoretical calculations based on comparing chemical structure to other materials of known Hildebrand value.