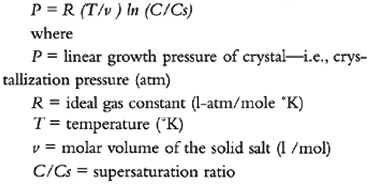
7 6. DETERIORATION MECHANISMS

There is no question as to the damage induced by the presence of salts in porous materials. The problem lies in explaining why and how damage occurs. The earliest postulated theories (Correns 1926; Correns and Steinborn 1939; Correns 1949) regarded crystallization pressure as responsible, and two mechanisms were considered: hydrostatic crystallization pressure and linear crystal growth pressure. Thirty years later, a thermodynamic model was developed for calculating crystallization pressure (Wellman and Wilson 1965). Hydration pressure was first suggested by Mortensen (1933) and has only recently been challenged. These theories and other proposed mechanisms are discussed in the following subsections.

7.1 6.1 CRYSTALLIZATION PRESSURE

Hydrostatic crystallization pressure can develop when a supersaturated solution occupies a smaller volume than the precipitating crystals plus the residual saturated solution, as is the case for most common salts (Correns 1926). Such pressure is directly proportional to the supersaturation ratio (C/Cs)—i.e., the ratio of concentrations of the solute in the supersaturated (C) to the saturated solution (Cs). However, when a pressure develops, the solubility of the salt also increases, according to the Le Chatelier principle, thus diminishing the supersaturation ratio. Therefore, as Duttlinger and Knöfel (1993) point out, this type of mechanism cannot account for the observed deterioration of porous stones by salts.

Linear crystal growth pressure and the equation developed for this mechanism are propounded in a widely quoted English paper by Correns (1949). The equation is:

Equation 1.

Although G. Amoroso and V. Fassina (1983) give a good discussion of crystal growth under these conditions, they fail to point out, as Duttlinger and Knöfel (1993) do, that the equation is applicable only for low supersaturation ratios, which would not result in sufficiently high pressures to deteriorate the stone. Correns's data showed significant deviation from the theoretical values for supersaturation ratios above 1.2. The frequently cited crystallization pressures (Winkler 1975) were calculated from high supersaturation ratios (2–50) that are highly unlikely in the pore systems of a stone. A comprehensive review and discussion of this topic has been presented by Duttlinger and Knöfel (1993). Another model that attempted to include this equation (Weyl 1959) is also discussed by C. Rodriguez-Navarro and E. Doehne (1999).

A second equation for calculation of crystallization pressure was developed from a thermodynamic model by H. Wellman and A. Wilson (1965, 1968). The model predicts that crystallization will originate in larger pores, as observed by Zehnder and Arnold (1989), and that porous materials with large capillaries connected to smaller ones are the most susceptible to damage. The pressure that builds up between two such connected pores as crystallization takes place is given by the modified Laplace equation:

Equation 2.

B. Fitzner and R. Snethlage (1982) applied this equation to calculate crystallization pressures for four German sandstones whose pore-size distribution had been measured. Results showed that pressure should increase uniformly with diminishing pore size, in contrast to differences in actual behavior of the stones. Using pore-geometry models, they developed two different multiplying factors, V1 and V2, to correct the calculated pressures. The one that gave the best results in comparison to results of actual crystallization tests proved to be:

Equation 3.

A similar approach was used by R. Rossi-Manaresi and A. Tucci (1990, 1991) to calculate the resistance to salt deterioration of different stones: three biocalcarenites, tuff, marble, and sandstone. It is interesting to note that these authors use the first correction factor, V1 = Vr/VR, which, in the case of the German sandstones, had not coincided with the experimental results. The calculations use changes of pore-size distribution in different samples of the same material—e.g., surface vs. interior. For example, the surface crust of the sandstone has a higher number of smaller pores, and hence the higher crystallization pressures should lead to its flaking. The heterogeneity in pore-size distribution of the calcarenites is postulated to produce an alveolar deterioration pattern. Finally, marble, which has few small pores, should be relatively resistant to salt crystallization. Nevertheless, Rossi-Manaresi and Tucci (1990, 1991) did not compare calculated values to actual experimental results.

7.2 6.2 HYDRATION PRESSURE

The pressure developed by volume increase upon hydration of a salt was postulated by Mortensen (1933), who proposed an equation similar to the previous one to calculate the hydration pressure:

Equation 4.

Of the salts that are capable of hydrating, none has received so much attention as sodium sulfate because of its highly deteriorating action on stone and its use in tests to evaluate durability of stone (Price 1978; McMahon et al. 1992). Many laboratory experiments have been carried out to try to determine whether its effectiveness as a deterioration agent could be attributed either to hydration or to crystallization pressures (Chatterji et al. 1979; Sperling and Cooke 1980, 1985). Further studies on the hydration-dehydration mechanism of this salt (Charola and Weber 1992; Doehne 1994) have shown that while the dehydration of the decahydrate, mirabilite, proceeds via multiple dissolution steps ending in a polycrystalline mass of submicron particles, the hydration rate of the anhydrous salt, thenardite, is slowed down due to the formation of a hydrated film on the crystal surface. In brief, hydration of this salt does not proceed via absorption of moisture by a solid crystal but only by dissolution of that crystal and reprecipitation from solution.

7.3 6.3 OTHER MECHANISMS

J. Pühringer (1983b, 1986; Pühringer et al. 1985) suggests that other mechanisms can also contribute to the deterioration of porous materials by salts. Among them he suggests that when salt films form on the walls of pores, shear stresses can result due to changes of temperature and humidity. As Duttlinger and Knöfel (1993) point out, this situation is unlikely, because in general crystals are not directly attached to the pore wall or support. Rather they are separated by a thin solution film. However, Correns and Steinborn (1939) described how some alum crystal faces {100} grew strongly attached to glass, while other crystal faces {111} did not adhere to it. This finding may be attributed to differences in phase-boundary energies that determine whether a solution film will remain between the two materials. It is possible that some crystals could adhere to a pore wall and exert stresses on it, mainly by temperature variations, but these can at most be a minor contributing factor to the overall salt deterioration process.

S. Lewin (1990) proposes that the effectiveness of salts as deteriorating agents is related to their ability to supersaturate. He considers that when a pore space is filled by a crystalline agglomerate, the crystals themselves are most likely imperfect. Therefore it is possible for ions to diffuse into the deposit and add themselves to interior sites. This process, however, requires mechanical work, and the energy for this can be provided only if deposition occurs from a supersaturated solution. No energy can be provided by crystallization from a saturated solution since the free energy of the salt in both states—solution and solid—is the same. Lewin exemplifies this theory using marble as the porous material and a supersaturated sodium chloride (NaCl) solution (7M). Although he stresses the importance of the tendency to supersaturation that different salts have with regard to their potential as effective deteriorating agents, the ranking he presents of the supersaturation tendency of the most common salts does not conform to observed deteriorating capacities.

Finally, Snethlage and Wendler (1997) have proposed a model of dilatation and contraction under the influence of moisture and dissolved salts to explain the deterioration of clay-containing sandstones. The model considers that any deterioration process, be it physical, chemical, or biological, changes boundary surfaces that will affect intergranular contacts. Thus, the original micromorphology will be altered (Snethlage et al. 1996). The relative displacement of stone grains due to changes in moisture and the effect that dissolved salts exert on this displacement enlarge smaller pores, providing more space for salts to crystallize in, as discussed in the following section. Snethlage and Wendler (1997, 15) suggest that “The idea of a crystallization and hydration pressure should therefore still be questioned, and further research should be carried out to verify the existence of these processes as damage factors.”

7.4 6.4 FURTHER LABORATORY EXPERIMENTS

Zehnder and Arnold (1989) developed a laboratory experiment designed to grow the different crystal forms observed in situ. For this purpose they used solutions with low supersaturation ratios of various salts, including sodium chloride, sodium nitrate, sodium sulfate, and magnesium sulfate, to grow crystals on both porous and nonporous substrates. They report that the most complete crystallization sequence for a given salt was obtained at a relative humidity just below the equilibrium RH, and they differentiate four crystallization stages. These stages were essentially similar for all tested salts. Initially, crystals of nearly the equilibrium form grew within the solution and preferentially in large pores. In a second stage, as the crystals and aggregates kept growing, still covered by solution, sufficient pressures were generated to disrupt the fabric upon which they grew. This development usually occurred where pores were close together or along discontinuities in the material. In the third stage, fissures induced previously accelerated the evaporation of the solution, and, as the material dried out, fibrous crystals started to grow. During the last stage, the fibrous growth turned into thinner whisker growth that could lift flakes previously detached from the surface. Arnold and Zehnder (1990) bring together the above experiments and their previous practical experience in a second excellent summarizing paper.

Rodriguez-Navarro and Doehne (1999) developed similar crystal growth experiments but using saturated solutions of sodium chloride and sodium sulfate to try to elucidate the reason for the differences in deterioration effectiveness of these two salts. They carried out micro- to macro-experiments, and one of the most interesting results is the crystallization of these salts in capillary tubes. While sodium chloride (halite) crystallized at the air-solution interface as well as within the solution, sodium sulfate decahydrate (mirabilite) crystallized only within the bulk of the solution. In the micro- and macro-experiments it was found that the growth rate of halite crystals was much slower than that of mirabilite. The different crystal shapes obtained for halite—ranging from perfect cubic crystals when grown within the solution, to hopper-shaped or dendritic growth when formed at the air-solution interface—agree with results obtained by Zehnder and Arnold (1989). Both mirabilite and thenardite, anhydrous sodium sulfate, grew as prismatic crystals within the solution at high (65%) and low (35%) RH, respectively. The distribution of the salts within the pores of the stones also differed. Halite filled smaller pores, while both mirabilite and thenardite filled the larger pores.

Another interesting experiment developed by C. Grossi et al. (1997) takes into account the polymorphism of the anhydrous sodium sulfate, of which five phases are known. Phase V is the familiar thenardite. In the experiment, acoustic emissions were measured, particularly in larger-pored stones, that indicated stresses in the stone were induced by phase transitions since the stone had dried out completely (@ 60°C) during the test. Smaller-pored stones retained some moisture and mirabilite crystallized during the cooling stage, but no acoustic emission was obtained. Although the test temperature is above that normally found in historic buildings, the phase-transition stresses for thenardite might have relevance for rock weathering in desertic areas.