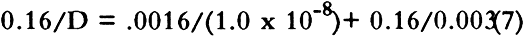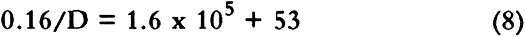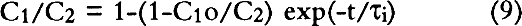4 RESULTS AND DISCUSSION

4.1 EFFECT OF SO2 CONCENTRATION ON DIFFUSION CONSTANT

The diffusion constants for two types of NARA boxboards at different SO2 concentrations are summarized in table 3. Data from sample A and sample B suggest that there are only small differences in the diffusion constants over a range of gas concentrations of more than a factor of 14.

These results suggest that the limiting low-concentration diffusion constant of SO2 in these boxboards may be close to the values of the diffusion constant we have measured. Thus, these data and previously mentioned observations tend to provide confidence in our experimental approach to this study.

4.2 EFFECT OF COMPOSITE NATURE OF BOXBOARD ON DIFFUSION CONSTANT

Many of the boxboards used to make boxes for archival storage are composite boards made of thinner boards glued together. This section is a discussion of the effects of the construction of the boxboard on the flow of SO2 through the boxboard.

Two extreme cases may be considered. In one case, the glue adheres to the boxboard matrix without filling the pores and impeding diffusion. In the other case, the glue creates a well-defined, intact polymer film with no holes between the two paperboards. In the first case, SO2 flux through the boxboard is not affected by the presence of the glue. In the latter case, the glue may completely control the flux.

In this study we have measured the diffusion across some boxboards that are composites made from other boxboard materials we have also studied. One such material, sample H, is a composite board manufactured by gluing three layers of sample F together. If the glue layer has no effect on the permeation properties, then the diffusion constant of samples H and F should be identical. Inspection of table 4 shows they are very different. By using information from the manufacturer of the board along with our data, we may try to estimate the effect of the glue layer on the diffusion.

The manufacturer of the boxboard claims that there is about 4.5 kg of dry adhesive deposited per 280 square meters of boxboard laminated. Assuming the density of the dry adhesive is one g/cc, we estimate a total thickness of adhesive of 0.0016 cm for the two glue films between the three pieces of boxboard.

For a composite board, the diffusion constants may be estimated from the diffusion constant of its components as (Crank 1975):

Fig. .

Fig. .

Fig. .

Fig. .

When equation 8 is solved, the diffusion constant for the composite board, sample H, is estimated to be 1.0 � 10-6. This value is within our estimated bound for the diffusion constant of sample H given in table 4.

The reader should note the dominance of the first term on the right-hand side of equation 8. This indicates that pollutant diffusion through the composite board is limited by the diffusion through the adhesive layer. Even an order of magnitude decrease in the boxboard diffusion constant would not change the observation that the adhesive layers control the flow through the composite boxboard.

If the glue film is not intact, however, the value of lf/Df could decrease by many orders of magnitude. This loss of total coverage by a film could arise in the original application and drying of the film or could result from the aging of the film. This variation in film coverage may explain why some composite boards have relatively high diffusion constants (samples A, B, and C), and some have very low diffusion constants (samples G, H, and J). Thus we believe the properties of the glue used in the manufacturer of the boxboard should be considered when estimating the overall flux of pollutants through a boxboard whose diffusion constant has not been directly measured.

4.3 COMPARISON TO EARLIER DATA

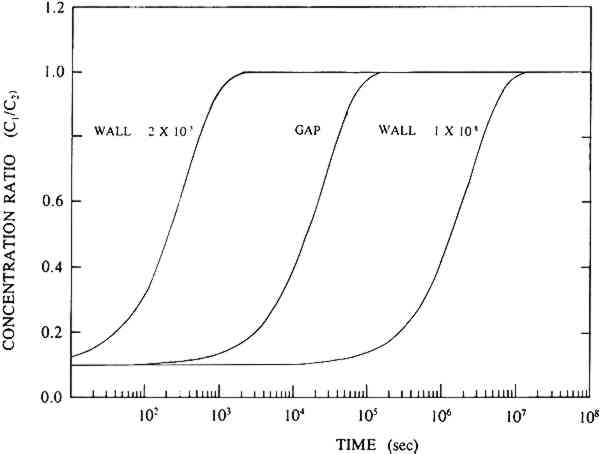
Dimitroff and Lacksonen (1986) reported a value of 0.007 cm2/sec for the diffusion of sulfur dioxide in air through stacked layers of rag paper. Their value of diffusion constant is higher than any value of diffusion constant obtained on boxboard in our study. There may be a variety of reasons for this difference.

First, Dimitroff and Lacksonen studied paper. The structure of the paper may be different enough from that of the boxboard to cause a difference in the diffusion constant.

Second, Dimitroff and Lacksonen made their measurement on a stack of paper. Between each sheet is an air space through which SO2 diffuses faster than it does in paper. Thus, their measured value of diffusion constant of SO2 in rag paper may be higher than the true value.

Finally, Dimitroff and Lacksonen measured the diffusion constant by sealing the edges of their paper. This boundary condition led them to measure flows through the paper that would be higher than would be measured in an infinite sheet. Barrer et al. (1962) modeled the diffusion constant measurement in a sealed system and showed the errors made by this measurement compared to the infinite sheet measurement (see our discussion in section 3.2). They show that the error in the measurement is strongly dependent on the ratio of the thickness of the sample to the radius of the cross-sectional area through which the gas is passing. As this ratio increases, the error increases. Our analyses of the Dimitroff and Lacksonen measurement system suggest that this ratio is about 0.4 for their measurements. In that case, the Barrer et al. (1962) study suggests the measured flow would be too high by at least 40% and would result in a high apparent diffusion constant.

4.4 COMPARISON OF EFFECTS OF DIFFUSION THROUGH THE WALLS OF BOX MATERIALS TO DIFFUSION THROUGH GAPS

Passaglia (1987) studied the microenvironment provided by a prototype archival box in protecting its contents from atmospheric pollutants. He presented a variety of models including: (1) a model that describes the protection provided by boxes that act as barriers to pollutants due to diffusion alone, and (2) a model in which the boxboard acts as a barrier both by reacting with the pollutant and slowing the flow of the pollutant through the boxboard. His models included calculations for prototype boxes filled with paper reactive with the pollutant and boxes that were empty. In all these models of the prototype box, he found that the pollutant flow into the box was affected by the flow through the gaps in the box arising from the construction of the boxes as well as the diffusive flow through the boxboard itself. His model separated out the contribution of the pollutant flow through gaps in the boxes as compared to the pollutant flow by diffusion through the boxboard itself.

Passaglia's models show a range of protection time scales. In the models that do not allow for reaction of the pollutant with the box material, he found that the time of protection is about a week. From the point of view of archival storage, the box allows for buffering of the materials in it only from short time variations in the pollution concentration. In the models in which he allowed for reaction with the boxboard and the paper filling the box, he found that the box may protect the contents for about a year. This time scale approaches archival storage time periods.

All Passaglia's (1987) models require an estimate of the diffusion constant through boxboard as well as other reaction constants of the pollutant with paper or boxboard. At the time, he had no direct estimate of the diffusion constant of SO2 through paper. He estimated the diffusion constant of pollutants through boxboard as 10-8 cm2/sec. With this value of the diffusion constant, in all his models he concluded that the mass flow of pollutant brought about by diffusion through gaps is much higher than the mass flow from diffusion through the boxboard itself. This conclusion suggests that closing the gaps will improve control of the microenvironment for any of those models.

From the measurements provided in this study, we find diffusion constants ranging from 10-3 to 10-6 cm2/sec for the various measured boxboards.

As an example of how this change in number can affect the conclusions to Passaglia (1987), the calculation for one of Passaglia's models was performed. In this model Passaglia considered the flow change due to diffusion alone. This model included no modeling of the reaction of the pollutant with boxboard. We employed this model because: (1) our work is mainly concerned with the diffusion constant alone, and (2) the effects of changing the diffusion constant on the other models follow a pattern similar to that found for this model.

In this model, the SO2 in the area outside the box is held constant at concentration C2. We consider a box that allows SO2 into the box due to diffusion through the boxboard and diffusion through the gaps. No reaction with the walls is considered. The box is considered to be empty. For this model, following Passaglia's equation 7, the ratio of the concentration on the inside of the container, C1, to the concentration of pollutant outside the container, C2, for flow through either gaps or through the boxboard is given by

Fig. .

Fig. 2. Ratio of C1, the interior concentration of an archival box, to C2, the concentration outside the box, as a function of time, for an empty box for (1) flow through gaps, (2) flow through box walls where the boxboard has a diffusion constant of 10-8 cm2/sec, of (3) flow through box walls where the boxboard has a diffusion constant of 2�10-3 cm2/sec.

TABLE 5 PARAMETERS USED TO MODEL PROTOTYPICAL EMPTY CONTAINER

With the value of the diffusion constant for boxboard in the range of the NARA boxboard value, we find that mass flow due to diffusion through the boxboard walls of the box is faster than the mass flow through the gaps. Thus, the use of boxes without gaps is of no importance for boxes made of these boards. However, for the boxboards with much smaller diffusion constants like those values found for boards G, H, and J, there may be some advantage to sealing the gaps.