DETERIORATION OF SURFACES EXPOSED TO ENVIRONMENTAL CHANGESGUSTAV A. BERGER, & WILLIAM H. RUSSELL
ABSTRACT—This research into the mechanical behavior of fabric-supported paintings goes back to the late 1960s when G. Berger's investigations culminated in the development of a new lining system for paintings (BEVA) (Berger 1972, 1975). Lining of the Atlanta Cyclorama required further research, which, since 1980, has been done jointly with W. H. Russell (Berger 1981). In order to measure the responses of canvas paintings to controlled changes in the environment, the authors have built a Biaxial Stress Tester for Stretched Canvas, described under “Test Equipment.” Numerous samples of old and new, painted and unpainted linen canvas have been tested under controlled environmental conditions of 0–50�C and 5–100% RH, and their stress changes have been recorded (Russell and Berger 1982; Berger and Russell 1984, 1986, 1988). The resistance of the samples to controlled dimensional changes (Modulus of Elasticity, “E”) has also been measured within the limits of the above environmental conditions.Since June 1984, a wealth of information has been collected with the help of automated data logging. Based on these findings, a working model of the decay of canvas paintings was developed (Berger and Russell 1984, 1986). Since all outer surfaces of solid bodies may be considered stressed membranes, these investigations have provided new insights into the process of aging and decay of all surfaces exposed to environmental changes. Investigations into the effects of some artists' techniques and certain conservation treatments have already been published (Berger and Russell 1986, 1987, 1988). Because not all of the above publications are readily available, they have been summarized in this paper, supported by actual examples and accompanied by practical applications (Berger 1983, 1984). Recent findings were incorporated to update, confirm, and elaborate on previously published material wherever necessary. 1 INTRODUCTIONPRIOR TO these investigations, it was impossible even to estimate the forces created by shrinkage or expansion of the different layers of a painting. The plasticity of the materials involved causes them to be continuously modified and transformed by their own responses to mechanical stress and/or environmental changes. Therefore, in order to repeat or compare stress measurements of polymers with any degree of accuracy, one must know the previous history of exposure of the measured materials (Dwan 1987; Guilly, Sotton, and Chevalier 1981; Kuzmitch 1978). For this reason, some of the initial results of this research were hard to interpret, as long as the measurements were recorded manually and had to be interrupted at night. With the introduction of continuous, automated data logging, the investigations took a giant step forward. Feedback of the electronic equipment made it possible to repeat the responses of the tested materials to environmental changes and to demonstrate the variations in reactions that occur with every cycle. Thus, some of the forces that lead to the physical decay of paintings, and possibly to the decay 2 MEASUREMENTS OF CANVAS TENSIONCHANGES OF up to 1500% in the tension of a stretched fabric in response to environmental fluctuations have been recorded by our stress tester. These changes have occurred in a sample drawn from a rather light canvas (linen, basket weave, commercially sized, and primed with acrylic paint, ca. 210 g/m2, 15 threads/cm in the warp (MD) and 18 threads/cm in the weft (CD) (fig. 1). It is likely that even stronger stress changes will occur in heavier canvas. Such changes take place in response to environmental fluctuations caused by daily or seasonal changes or by cycling environmental devices. The responses of stretched canvas to environmental changes vary greatly. In the canvas described in figure 1, the tension rises with a rise in relative humidity (RH). Another canvas (linen, basket weave, commercially glue-sized, and primed with oil paint), shown in figure 2, has its peak tensions at periods of low RH, although it looks very similar to the one in figure 1. In fact, every canvas measured in our tests responded differently to changes in RH and temperature, although all the canvases were linen, and these responses often changed gradually during testing.
∗ See General Remarks at bottom of page 48
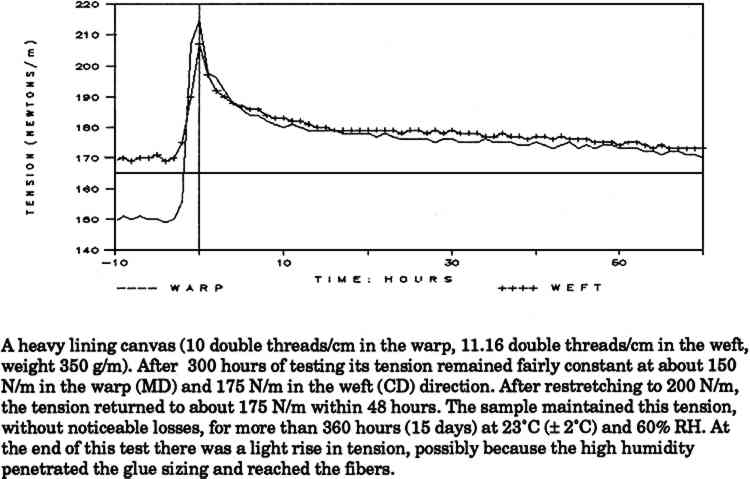
∗General Remarks about Figures 1, 2, 11, and 18. The top diagram (a) shows changes in temperature and RH. The second diagram (b) shows the changes in tension in response to the environmental fluctuations recorded in (a). Wherever there is a third diagram (c), it shows the values in a different vertical scale (y) in order to facilitate comparison with other diagrams in the same scale or to provide a clearer picture of smaller changes. In view of these findings, it is impossible to assign a single value of tension even for a specific canvas. Instead, we found that each stretched canvas has a wide range of values in response to combinations of temperature and RH. When attempting to assign a measure of tension to a certain stretched canvas it is therefore necessary to use one of the environmental conditions as a benchmark. The generally accepted “normal” conditions of 21�C and 60% RH were chosen arbitrarily, since for most sized and painted canvases the tension at these environmental conditions is near their lowest point. Henceforth, when referring to canvas tension without specifying environmental conditions, we mean the tension at 21�C and 60% RH. Tension stress is given in Newtons per meter; 100 N/m corresponds roughly to 0.5 lb of tension per inch, or 98.1 g/cm (ca. 10 kg/m). Most conservators would consider this a fairly strong tension and, indeed, this is about the maximum tension an average canvas can sustain for any length of time. 3 STRETCHING A CANVASWHILE STRETCHING a canvas, we intuitively reach a certain limit of tension, which is set by the fact that the canvas would tear at the tacks if stretched any further. This tension is usually around 200–300 N/m (l lb/in). When we check this canvas a few days later, we find that it has slackened and lost about one half of its tension (Hedley 1988). We can hasten the slackening of canvas by wetting, which would initially raise the tension to 700–900 N/m typically and overstretch the canvas in the process (fig. 1). Following drying, the tension drops to 40–60 N/m. No matter how often such cycles of stretching and wetting are repeated, the result will always be similar. Although there is usually a slight rise in the end-value at all points of the tension, caused by “case hardening” (i.e., polarization of the fibers) (Guilly, Sotton, and Chevalier 1981), the tension nevertheless reverts to almost the same level (fig. 3). We call this value maximum sustainable tension (MST). This value is valid, however, only for a specific environmental condition, as, for example, 21�C and 60% RH. From the 100th to the 180th hour of the test of the canvas described in figure 1, a steady MST of 200 N/m at 60% RH is recorded. When the canvas is stretched to 200 N/m, it has specific tensions for each level of temperature and RH. In
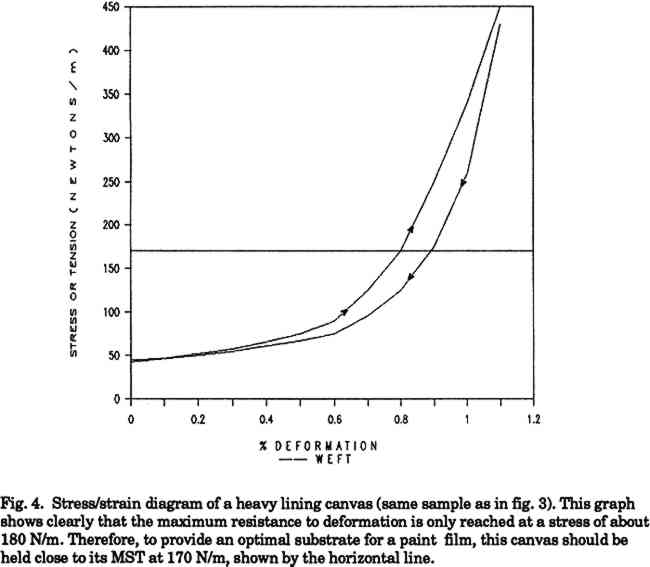
This brings out an important fact: The exposure to high RH and the resulting rise in tension to over 800 N/m have permanently deformed and enlarged the canvas. Unless the canvas is restretched (keyed out), it will no longer reach the tension of 100 N/m at 20% RH or of 200 N/m at 60% RH. This is the effect of only one short exposure to high RH (about three hours). Our tests have shown that longer and repetitive exposures to high RH lead to even more severe tension losses. Once stretched out, canvas can never be pushed together again by mechanical means. The environmental conditions to which the stretched canvas was exposed have caused irreversible mechanical and dimensional changes. These changes have resulted in a loss of tension which can only be corrected by outside intervention, such as keying out. Canvas stretched on a rigid stretcher may be considered a mechanism that, under the influence of environmental changes, enlarges the canvas and produces a loss of tension. It must be noted that as long as tension is maintained, the actual physical dimensions of the stretched canvas do not change. While we understand that the loss of tension is due The authors have repeated this experiment several times with the same results (Russell and Berger 1982), as did Guilly, Sotton, and Chevalier (1981). Depending on the canvas, it takes a size increase of about 0.3–0.4% to achieve the rise in tension necessary to regain the initial 205 N/m. However, for the “real” measurement of the change, this expansion is not enough, since with any sudden increase in tension there occurs slippage of the fibers and creep and the resulting rapid loss of tension. Repeated measurements have shown that a stretched canvas loses 30–50% of its tension in the first 48 hours following stretching (see above and fig. 3) (Russell and Berger 1982; Hedley 1988). To regain the initial tension of 205 N/m (the MST) after 48 hours, we would have to stretch the canvas by an additional 0.3–0.4% (a total of 0.6–0.8%) in order to raise the tension temporarily to above 300 N/m, which would allow it to drop to 205 N/m (after 48 hours). In a one-meter-long painting, 0.3–0.4% equal 3–4mm. Since keying out increases the size of the canvas in both directions, only one-half of this value is needed on each side (that is, square root of 3 to square root of 4, or 1.7–2.0 mm in each direction). Sizing, priming, and aging can change these values considerably. 4 THE IMPORTANCE OF TENSION FOR CANVAS PAINTINGSSTRETCHING A fabric is the easiest way to give a practical form to the idea of a perfectly even plane. Surprisingly, the enormous stress changes in stretched canvas have little effect on its actual size. With a good, strong stretcher, the change in the length of the yarns and in the size of the fabric itself is minimal, due solely to the contractions and expansions of the stretcher. If the stretcher does not move, the dimensions of the stretched fabric do not change. Under strong tension, a stretched fabric is consistently more elastic and resilient than a solid material of equal weight. As long as the fabric is kept under tension, it remains inert and flat. Thus, a stretched fabric is an excellent engineering solution for a large, flat, stable, and resilient surface of low weight and low cost. This accounts for its popularity as a painting support. 5 STIFFNESS, RESISTANCE TO DEFORMATION (MODULUS “E”)WHEN A fabric loses its tension, it also loses its elasticity and its resistance to deformation. Canvas under tension must be straight unless it is deformed by a stronger force. The reverse of aged canvas paintings provides clear evidence that the paint film deforms the canvas, not the other way around, as was widely believed. This and other considerations led us to test the stiffness, or resistance to deformation, of stretched canvas (Modulus of Elasticity, E) (fig. 4). It was measured in the same range of temperature and RH as generally used in our tests, that is, from 4% RH to 100% RH and from freezing to 50�C. These tests showed that within the above limits, temperature and RH had only very little influence on the resistance of canvas to deformation. Instead,
6 STRESS-VALVES OR DEFORMATION-TRAPSTHE TYPE of mechanism that leads to deformations and dimensional changes in materials exposed to environmental changes was first observed by Richard Buck (1978). He noticed that a panel painted on one side only is inexorably deformed by repeated cycles of humidity changes. In fact, it is deformed to such an extent that no single physical force could bring it back to its original condition. This happens through the one-sided accumulation of small dimensional deformations created by every rise in humidity. In Buck's example only compression is accumulated, while elongation is eliminated. In stretched canvas the opposite is the case. Our research has found many such one-sided layouts in the geometry of materials, which lead to the accumulation of deformations during aging. We have called these configurations stress-valves or deformation-traps because they permit only one type of stress, either compression or tension (Berger and Russell 1986). Figures 5 and 6 show schematically the most common stress valves that occur in paintings. The top diagram, for cracking, shows the paint film at rest (fig. 5a). There are two heavy layers of paint at both ends and a weak layer between them. Here the weak layer is shown as being thinner, but it could also be weaker for a number of reasons. The paint layer expands when the temperature rises or when exposed to solvents. This is a well-documented fact supported by ample evidence, some of which will be shown later. If the paint is not held firmly, the strong, heavy layers expand and compress the weak layer, which, as a result, becomes shortened (fig. 5b). In the subsequent cooling or drying cycle, the contraction of the paint shortens all parts of the paint film (fig. 5c). However, the weak portion of the paint film cannot resist the strong tension of the adjacent heavy layers. It becomes elongated against its own tendency to contract and is overstretched in the process. As a result, the overstretched part is further weakened and eventually ruptures. After the rupture, the paint film can only be compressed in the expansion cycle (fig. 5d). From now on, contraction will only open the crack, but no longer cause the film to elongate (fig. 5e).
Figure 6 (6a–6c) explains the circumstances that lead to blisters. Here, a failure of adhesion between the paint film and the substrate is shown by the open space between them. In figure 6b, a contraction leads to an overextension of the weak, unattached film. A subsequent expansion cycle (fig. 6c), does not compress the loose film, which evades compression by buckling. Thus every contraction elongates the unattached section, but subsequent expansion no longer shortens it. Cracks are a sign that the paint film has contracted and has become smaller because cracks take space. Blisters are a sign that the paint film has expanded since a bent paint film must be larger than a flat one. Both cracks and blisters can often be found side by side in the same painting. It would be hard to explain this seeming paradox in any other way than by the stress valves described above. Cupping of the paint film and warping of the canvas can be explained by similar mechanisms. We could safely generalize and say that such stress-valves can be found whenever aging causes physical or dimensional changes. They work like a pump: every environmental change is another stroke of this “pump,” which fills up the reservoir of stress or deformation. The elements that lead to the deterioration of surfaces exposed to environmental changes are as follows:
Repeated variations in temperature and RH (e.g., during day and night or due to thermostatically controlled devices, such as cycling air-conditioners and heaters, turning on or off electric lights, using radiating heat sources such as incandescent lights and the light coming through the windows), lead to the accumulation of the nonrecoverable dimensional changes. As a result, the materials fail, just like a wire when repeatedly bent back and forth. For example, in the case of paintings, the paint film cracks to avoid extension or blisters to avoid compression, depending on the location of the initial failure. 7 EFFECTS OF CYCLING ENVIRONMENTAL CONDITIONS ON PAINT FILMSTHE CHANGES of tension resulting from the expansions and contractions of the canvas can be measured directly by our stress tester. The effects of the glue-sizing become evident by changes in the patterns of the reactions of the sized fabric. The application of stiff paint—gouache or epoxy, for instance—causes equally dramatic changes, similar to the effects of glue-sizing. In addition, particularly with epoxy paint, the effects of thermal expansions and contractions become clearly visible. Pigments and polyester films are less susceptible to changes in RH than is cellulose, but they react to changes in temperature. On the other hand, a freshly applied oil paint film causes hardly any stress changes. Although initially disappointing, this is possibly one of the most important findings of our tests: A soft paint film does not develop any stress. If such a film is firmly attached to its substrate, it compresses readily when it expands and becomes thicker in the process. When it contracts, it simply becomes thinner again (fig. 7), just as a liquid held in a receptacle always stays in place, although it rises when expanding and falls when contracting. However, when a paint film becomes harder and stiffer than its substrate, it deforms the substrate by its expansions and contractions. The film begins to behave like a solid body or plate. In such a plate the expansions and contractions are proportional to the dimensions of the body in the direction in which they occur (fig. 8). The larger the plate, the larger the movements of its borders. Alternately, the larger the plate, the higher the stress concentrations in its weak spots when its borders are restrained.
Sometimes the edges of such plates are only partially restrained, as for instance, in a modern painting with areas of heavy paint surrounded by thinly painted canvas (fig. 9). In such a case, the central elements of the heavy plate will have to overcome the resistance of the surrounding areas. When the paint expands, the central part will have to push against the expansions of all the surrounding paint and the resistance of the canvas. When the paint film contracts, the central part will have to pull all the combined tensions of the rest of the plate. Thus, a plate that can move on its substrate multiplies and concentrates the stress generated by all its parts.
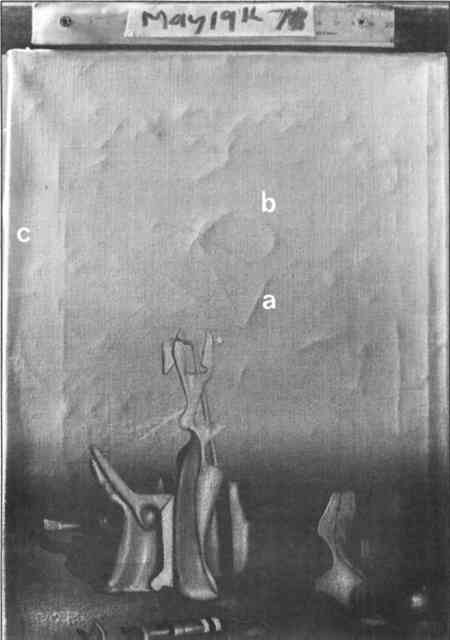
In a painting that is 30 to 50 years old, as in the interior by Alice Stoddard (detail, fig. 10) the first cracks develop in the center of the largest and hardest “plates” of heavy paint. At the same time, thinly painted areas stay free of cracks because they form no plates and, therefore, cannot generate the forces necessary to crack a paint film. Of special interest in fig. 10 are also the concentric cracks surrounding the area of heavy impasto,
At low tensions the effect of the thermal expansions can easily be observed on the stress tester. This is shown in figure 11, another diagram of the “shrinking canvas,” first described in figure 1. In a badly prepared canvas, such as this one, values of the MST (see “Stretching a Canvas”, above) are well below the elastic limits of the canvas. Therefore, under certain environmental conditions, the canvas has no longer sufficient stiffness to support the paint film adequately. The stress diagram in figure 11b is shown in enlarged scale in figure 11c. Between the beginning of the test and its 15th hour, and again at its 48th hour, there are large stress fluctuations caused by changes in temperature. These changes cannot be explained by the behavior of canvas, but are indicative of the expansions and contractions of the paint film. They occur only at changes in temperature. Indeed, the large rise and fall in relative humidity, from 20% to 50% and back, at the 37th and 53rd hours, do not cause as large a stress change as the fluctuations in temperature of less than 5�C at the 12th and 48th hour. These stress changes have an amplitude of 80 N/m,or more than 100% of the lowest tension recorded in this test. At these low tensions a fluctuation of 80 N/m indicates a movement of more than 0.3%, which is quite a considerable strain. Changes in temperature of 5�C are frequently repeated in rooms equipped with cycling heaters and air-conditioners. They activate the mechanism of decay described by the stress-valves and probably are the main cause of the damages previously attributed to their drying effect alone.
∗ See General Remarks at bottom of page 48 8 CYCLING ENVIRONMENTAL CONDITIONS AND DISCONTINUITIES IN THE STRUCTURE OF THE PAINTINGCANVAS UNDER stress adjusts until every yarn tends to carry an even load over all of its length. If, for some reason, certain yarns receive a bigger or smaller load than the others, they expand or contract as need be until an equilibrium is regained. The plasticity and creep of textile yarns give them the capability to adjust. This capability makes it possible to stretch canvas into a perfectly smooth plane, as opposed to a stiffer metal screen or fiberglass fabric, which lacks the necessary “give.” Plasticity and creep also make it possible for fabric to regain this plane whenever it is disturbed. This is not the case with metal plates, which once deformed (dented) cannot be straightened out without loss of paint, or with wooden panels, which warp. In contrast, the softness of slack canvas makes it possible to bring deformed canvas paintings back into a nearly perfect plane surface without loss of paint. The plasticity and creep of fabrics play an important role in their performance as supports for paint. This is why canvas paintings could be kept flat for centuries. A stretched membrane (fabric) will maintain a plane surface only as long as it has a uniform or symmetrical cross-section (fig. 12a). Priming and painting increase the cross-section on one side of the fabric. Applying paint locally (or cracking of paint) alters the uniform cross-section of a fabric (fig. 12b). Initially the paint film is soft and has little effect on the expansions or contractions of the fabric. However, as paint ages, its stiffness increases, and it begins to resist stress. If the tension in a partially painted fabric
This basic principle of stress propagation was first used by G. Berger to explain deformations in canvas paintings, such as cupping and bulging, as early as 1972 (Berger 1972, 1975; Skeist 1965), and later demonstrated at professional meetings (Berger 1978, 1980). Stress propagation is one of the main causes of cupping in fabric-supported paintings, though other factors common to all surfaces exposed to environmental changes also contribute to it. After all, cracking and cupping are not confined to canvas paintings. These factors are the compacting and shrinkage of the paint film by cross-linking of the exposed top surface (Berger 1980) and the cumulative compression by cycling stress changes (Berger and Russell 1986). Any of these additional distortions can be reduced by relaxation and vapor treatments. However, all the above contributing factors stem from the same defect, namely, the one-sided interruption of the line of stress, which cannot be eliminated by relaxation of the cupping alone. Discontinuity of the stretched surface by heavy pastose paint films, cracks, tears in the fabric, patches, or glue concentrations must lead to distortions in the plane of the painting. Effective lasting treatment can only The small oil on canvas by Yves Tanguy (fig. 13) was disfigured by strong local cupping, which greatly impaired its “readability.” This cupping was produced by minute breaks caused by the design the artist scratched on a commercial priming that was probably already several years old at the time the artist painted on it. Later handling and a customs stamp must have created further breaks in the priming. These breaks formed “deformation valves” that, because the painting was in a private collection and exposed to frequent cyclic changes, produced considerable cupping in an otherwise well-preserved paint film. In a young painting such as this, where the priming and paint film are still relatively soft, such deformations can be easily corrected by heat and vapor treatments. If, following this treatment, the painting is kept in a very stable environment—as, for instance, in an air-conditioned museum—the cupping might not return because the deformation valves would not be reactivated. However, were the painting to return to the same uncontrolled environment, the cycling changes would reactivate the deformation valves and cupping would reappear. The recurrence of the cupping would be more rapid than before, because the paint film has become stiffer due to aging and the discontinuity in the stress distribution is now larger.
Other stress differentials and discontinuities of stress develop between parts of the painting that are protected from changes in the environment and those that are not, thus resulting in cracking (fig. 14)(Russell and Berger 1982; Urbani 1973). Consequently, the first cracks usually develop along the edges of the stretcher (“stretcher marks”). Our measurements show that small changes in temperature and RH create considerable differences in tension (fig. 11). The areas of the painting protected by the stretcher lag behind in their reactions to outside conditions and, as a result, a difference in tension between these areas and the rest of the painting is created. One part of the painting reacts more than the other, and a fault line develops between the exposed part of the painting and the area protected by the stretcher. The cycling changes are more severe in the unprotected part, and the paint starts to crack, proving that protection from cycling environmental changes is one of the most important measures a conservator can take to prevent or, at least, retard the process of decay of paintings, glass, wood, plastic, and even stone monuments.
9 PROTECTION OF PAINTINGS UNDER ADVERSE ENVIRONMENTAL CONDITIONSIT IS rarely possible or economically feasible to protect paintings from all possible changes in the environment. Indeed, it could be said that many paintings have kept quite well without any environmental controls. Most paintings are quite tough, and paint has been used to protect other surfaces from decay since ancient times. Moreover, some paintings crack or blister profusely over part of their surfaces, while other parts of the same paintings that are exposed to the identical environment remain in perfect condition. It follows that certain conditions innate to the painting have protected it from deterioration. As long as an oil or an acrylic painting is “young” and flexible, it keeps quite well under
10 PRACTICAL EXAMPLES SUPPORTING THE NEW MODEL OF PAINT DECAYONCE THE rules of surface decay became clear to us, we found overwhelming evidence to support them in paintings we inspected. To keep this article within manageable limits, only some of the most striking examples are given below to prove the importance of a stiff support for the preservation of paintings. In addition to the painting on copper (fig. 15), the Austrian or Czech late Baroque sketch, 56 � 43.5 cm. is an excellent example (fig. 16). Like many early canvas paintings, it was fastened to its strainer with glue rather than nails. The strainer, made of solid lindenwood, could not be keyed out. Because of its expansions and contractions, the canvas within the strainer has slackened and distorted. It was, therefore, decided to remove the painting from its strainer. Regrettably, we did not realize at the time that we had an excellent example for our theory. The 4 cm–wide strip where the painting was firmly glued to the strainer was free of cracks. However, the unattached area in the center was covered by the usual primary and secondary crackle system, typical of a 250-year-old canvas painting. Since the entire painting has the same technique, materials, and history, the excellent preservation of the 4 cm–wide edges can only be attributed to the firm support provided by its attachment to the strainer.
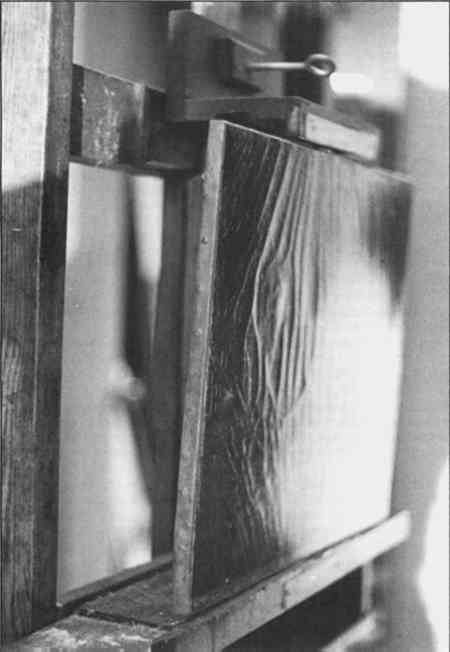
A discarded 17th-century copy of The Spinners by Vel�zquez was extensively tested in our lab (fig. 17)(Berger and Russell, 1987). Our tests determined that this canvas was six times stiffer in the vertical (weft) direction than in the horizontal (warp) direction. The photograph shows that most of the cracks run in the vertical direction. Therefore, the paint cracks in the weaker, more flexible horizontal direction of the canvas.
The stiffness of the canvas provided by constant tension also explains the amazing state of preservation of cyclorama paintings, which originally inspired this research (Berger 1981). The upper parts of these huge canvases are stretched by the weight of 12–15 meters of heavy, painted canvas pulling on them. This continuous tension supplies sufficient stiffness to resist the movements of the paint. It has protected the paint film from cracking and cupping, in spite of the very adverse environmental conditions in which these paintings were kept. In the bottom parts of the cycloramas, where there is no such tension, the paint has the cracks typical of a neglected, century-old painting. Based on the above observations, a new stretcher for canvas paintings was designed to simulate the beneficial effects of constant tension (Berger 1983, 1984). The spring-loaded stretcher is said to have been invented by Wright and Gardner in 1875, and a later one was designed at the Fogg Art Museum and subsequently used by Richard Buck and many others, including one of the authors. Other versions were developed by Rigamonti in Italy, Vidal in France, and others. However, all these designs with diagonal expansions in the corners were structurally unsound (wobbly) and ameliorated only partially the defects of the rigid stretchers, while introducing new problems along the diagonals. The self-adjusting constant tension stretcher (U.S. Patent Serial No. 571,350) follows an entirely new design. It has a rigid, structurally sound body and permits the canvas to expand and contract freely, in both directions, in response to environmental changes. The suspension system of the self-adjusting, constant-tension stretcher was repeatedly tested on the stress tester. Comparing the stress fluctuations of the “shrinking canvas” held on a rigid stretcher (up to 1500%) (fig. 1) with the same canvas suspended by springs (30%) (fig. 18), one can see clearly the benefits of the self-adjusting stretcher. The sample supported by springs is exposed to much more violent fluctuations of the environment, but its tension remains virtually constant. As a result, the paint has sufficient support at all times. In addition, while in figure 1 the tension dropped by 50% after a single exposure to 100% RH, the spring-supported sample shows no drop in tension even after two prolonged exposures to 100% RH. In addition, constant tension reduces cupping, since the stress differentials due to shrinkage and expansion of the canvas are eliminated. The painting Easter Monday, by Willem de Kooning (Metropolitan Museum of Art, New York), cracked and delaminated and was removed from display due to its precarious state. Following mounting on the self-adjusting, constant-tension stretcher, the condition of the painting has improved, and it is now on permanent exhibition. It even graces the cover of at least two of the museum's publications. We have received similar reports regarding paintings by Sam Gilliam and Helen Frankenthaler at the High Museum of Art in Atlanta and a rather large Morris Louis at the Philadelphia Museum of Art.
∗ See General Remarks at bottom of page 48 However, the constant-tension stretcher can only succeed if the canvas retains sufficient strength to support the tension exerted on it by the springs. This tension is usually smaller than the peak tensions imparted to the painting by its natural contractions. If the canvas is too weak to be stretched sufficiently to effectively support a brittle or uneven paint film, then lining is indicated. Not only does lining impart stiffness to the canvas, but by its bulk it also protects the painting from fluctuations of the environment. Figure 19 shows a painting by Jean L�on G�r�me that was patched or “locally lined” in the past, obviously before cracking set in. The stiffness of the patches has protected the patched areas, which are free of cracks, while the rest of the painting is severely cracked. Had the patch covered the entire painting, it would have remained free of cracks.
11 CONCLUSIONSOUR TESTS have shown that to protect canvas paintings from environmental changes, the following steps are required:
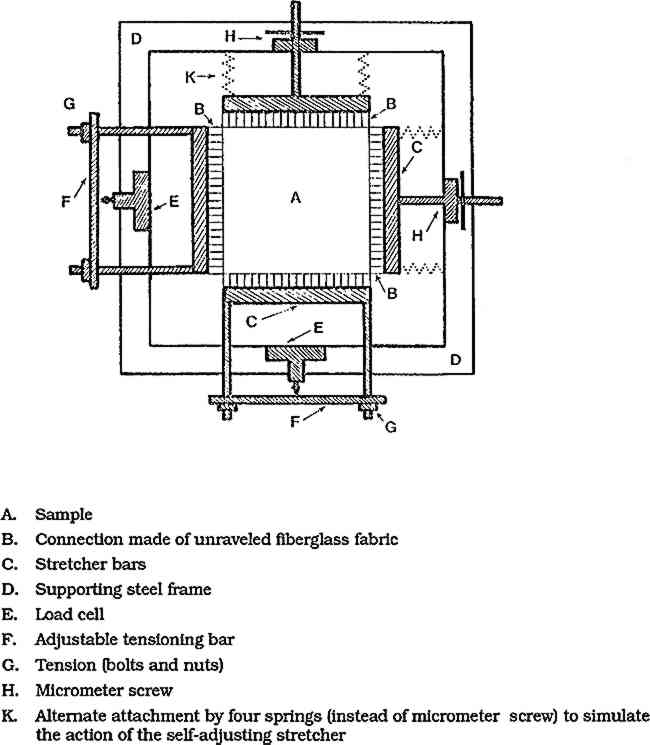
The continuous surveillance of the stress changes in the tested samples and the detection of alterations that occur in the material in response to environmental influences have provided an entirely new understanding of the mechanisms of aging and decay, not merely of canvas paintings but of all surfaces exposed to environmental changes. 12 SUMMARYIN TEN years of research, the authors have conducted extensive tests yielding important conclusions, practical applications, and publications on the responses of canvas paintings to environmental changes. Since the outer surface of every solid object may be considered a “stressed membrane,” these findings also have led to a new understanding of the process of decay and aging in general. 13 TEST EQUIPMENTA BIAXIAL stress tester for stretched canvas shown in figures 20 and 21 was built and perfected (Russell and Berger 1986; Berger and Russell 1988). The test arrangement, whereby the samples are subjected to controlled environmental changes, closely resemble the conditions to which canvas paintings are actually exposed. Canvas samples, 25 � 25 cm in size (A) are mounted within a rigid steel frame (D), which restrains the deformation of the canvas during testing. Threaded rods (G) permit adjustments of the tension in the canvas. The stress in the warp and weft directions is measured by separate load cells (E). Thus, it is possible to observe the restrained canvas sample and measure changes in tension (stress) as they occur in actual paintings. To ensure a uniform stress distribution, the edges of the sample must be supported continuously. Each direction also must be able to respond independently. These conditions are met with fiberglass fabric (B) whose
∗ Reprinted from Studies in Conservation 33(4):189 with the permission of the International Institute for Conservation of Historic and Artistic Works, 6 Buckingham Street, London, England WC2N 6BA. The testing frame is enclosed in an environmental chamber that makes it possible to subject the sample to changes of temperature (between 0�C and 50�C) and RH (from ca. 5% to 100%). The arrangement proved extremely sensitive. Changes of 0.1�C or less than 0.1% RH that could not be recorded on the measuring probes often caused measurable changes in tension. As testing continued, it became clear that the previous history of the environment had a considerable influence on the reactions of the sample. The stress tester was therefore automated to collect readings for extended periods without interruption. In 1984, Gordon Minns, President, Stable Technologies, Suffield, Connecticut, wrote a special program for data gathering. His program records readings every 15 seconds on a liquid crystal screen. They are printed on paper in selected intervals and recorded on magnetic tape. Once a week the data are transferred to disk, but during vacations the computer can work for months without supervision. As we became more familiar with computers and measuring instruments, we began to use feedback from the environmental chamber to produce recurring patterns of environmental changes that could be compared with each other. This system of controlled cycling is presently our main research tool in conjunction with biaxial stress/strain measurements of canvas, for which this experimental set-up seems very well adapted. ACKNOWLEDGEMENTSTHE AUTHORS wish to thank the Samuel H. Kress Foundation and its president, Dr. Marilyn Perry, for continuous financial and moral support of this research project. Grateful acknowledgment goes to the Georgia Institute of Technology College of Architecture for its continuous help. To the Brown Foundation, Inc., and the numerous other dedicated supporters, gratitude is expressed. The contents of this paper were presented at the 16th Annual Meeting of the American Institute for Conservation in 1988. REFERENCESBerger, G. A.1972. Testing adhesives for the consolidation of paintings. Studies in Conservation17:173–93. Berger, G. A.1975. Heat-seal lining of a torn painting with Beva 371. Studies in Conservation20:126–51. Berger, G. A.1978. Preventive conservation of painted objects. ICOM Committee for Conservation 5th Triennial Meeting, Zagreb, 78/6/1. Berger, G. A.1980. Decay of painted objects: Causes and prevention. In International symposium on the conservation of contemporary art, Ottawa: National Gallery of Canada. Berger, G. A.1981. The role of tension in the preservation of canvas paintings: A study of panoramas. ICOM Committee for Conservation 6th Triennial Meeting, Ottawa, 81/2/3, 1–12.
Berger, G. A.1983. Winning canvas paintings' battle of the bulge: A structural alternative. Technology and Conservation4(Spring 1983):5–8. Berger, G. A.1984. A structural solution for the preservation of canvas paintings. Studies in Conservation29:139–42. Berger, G. A., and W. H.Russell. 1984. The new stress tests on canvas paintings and some of their implications on the preservation of paintings. ICOM Committee for Conservation 7th Triennial Meeting, Copenhagen, 84/2/7–9. Berger, G. A., and W. H.Russell. 1986. Investigations into the reactions of plastic materials to environmental changes. Part I: The mechanics of decay of paint films. Studies in Conservation31:49–64. Berger, G. A., and W. H.Russell. 1987. Some conservation treatments in the light of the latest stress measurements. ICOM Committee for Conservation 8th Triennial Meeting, Sydney, 1:127–36. Berger, G. A., and W. H.Russell. 1988. An evaluation of the preparation of canvas paintings using stress measurements. Studies in Conservation33:187–204. Bresee, R. R.1986. General effects of ageing on textiles. Journal of the American Institute for Conservation25:39–48. Buck, R. D.1978. The behavior of wood and the treatment of panel paintings. In Upper Midwest Conservation Association, collection of papers 1952–1972. Minneapolis: Upper Midwest Conservation Association. Dwan, A.1987. Paper complexity and the interpretation of conservation. Journal of the American Institute for Conservation, 26:1–18. Guilly, R., M.Sotton, and M.Chevalier. 1981. Etude des propriet�s de doublages experimentaux � la colle synthetique. ICOM Committee for Conservation 6th Triennial Meeting, Ottawa, 81/5/1–13. Hedley, G.1988. Relative humidity and the stress/strain response of canvas paintings: Uniaxial measurements of naturally aged samples. Studies in Conservation33(3):133–48. Kuzmitch, L. A.1978. A new method of investigating stressed state of artistic works in restoration and conservation practice. ICOM Committee for Conservation 5th Triennial Meeting, Zagreb, 78/1/10. Russell, W. H., and G. A.Berger. 1982. The behavior of canvas as a structural support for painting. Science and Technology in the Service of Conservation, IIC-Washington Congress, London: IIC. 139–45. Skeist, I.. 1965. Handbook of adhesives. New York: Reinhold Publishing Co.78. Stolow, N.1977. The microclimate: A localized solution. Museum News55(November/December Technical Supplement):52–63. Thomson, G.1977. Stabilisation of RH in exhibition cases: hygrometric half-time. Studies in Conservation22:85–102.
Urbani, G.1973. Applicazioni delle “Termovisione” nel campo della conservazione delle opere d'arte. In Problemi di conservazione, ed.G.Urbani. Bologna: Editrice Compositori. 317–27. AUTHOR INFORMATIONGUSTAV A. BERGER studied art in Vienna, civil engineering at the Haifa Technical Institute, and art restoration in Bologna. In New York, he was assistant to Mario Modestini and William Suhr. In 1965, he began research on adhesives for the consolidation of paintings, supported by grants from the Samuel H. Kress Foundation. After developing a new adhesive, BEVA 371, he went on to investigate and develop other materials for use in conservation on a grant from the National Endowment for the Arts. Since 1980, his research has focused on the reaction of stretched canvas to changes in ambient temperature and humidity. He heads the Art Conservation Research Foundation, Ltd., a public, not-for-profit organization. Address: Art Conservation Research Foundation, Ltd., 115 West 73rd Street, New York, New York 10023-2940. WILLIAM H. RUSSELL has an MSAE degree in Architectural Engineering from the University of Illinois (1973). He taught at the University of Illinois, and has been Assistant Professor, Georgia Institute of Technology College of Architecture, since 1979. He is a member of numerous professional societies, including American Institute of Architects, American Institute of Steel Construction, and American Concrete Institute. Russell is a practicing registered architect and structural engineer. Address: Georgia Institute of Technology, College of Architecture, Atlanta, Georgia 30322.
 Section Index Section Index |